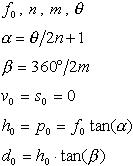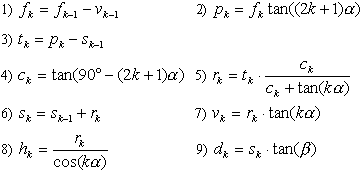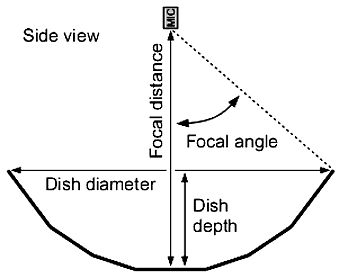
On this page, you can calculate dimensions of flat trapesoidal pieces which, when assembled together in a prescribed order, form a 3D object that approximates a paraboloid of rotation - which is just a fancy name for a more familiar thing we call "parabolic reflector".

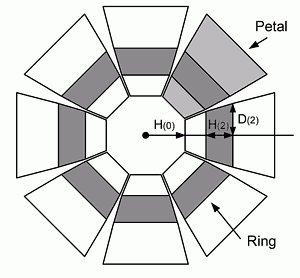
As can be seen in the image to the left, trapesoidal "cells" are arranged in a flower-like 2D shape. The smaller piece dimensions are i.e. the more rings and petals there are in the "flower", the smoother the final 3D paraboloid will be. It is however neither practical nor necessary to exaggerate the importance of smoothness as it is sufficient that trapesoids are not much larger than the wavelength of the highest frequency sounds one intends to collect with the reflector. For example, if the highest audio frequency in interest is 15 kHz, then the largest trapesoids outh not be much bigger than 340 m/s / 10 000 Hz = 3.4 cm. Tests show that reflectors up to 0.7 m in diameter perform quite well if there are not more than 8 rings and 16 petals in total. Although outer trapesoids in such reflectors will probably have more than a few centimeters in height or width, those ones nearer to the center are practically always small enough to render the reflector perfectly up to the task over the whole audio frequency band.

This calculator forms a reflector surface in such a way so that acoustical energy reflected from the center of each trapesoidal cell surface is directed precisely towards the microphone capsule mounted at the focal point, while energy reflected from outer points of each cell goes a bit around the focal point. If this were not so, energy reflected from most cells would be focused much closer and/or further than the focal length missing the microphone. Consequently, the reflector would suffer from heavy "spherical aberration" which means that focusing would be lousy as it would not be able to direct most of the available acoustical energy onto the microphone. This is exactly the reason why we don't want to use simple spherical reflectors instead of paraboloids.

A small animation on the left explains the intended method for drawing a "flower" shape after cell dimensions have been calculated. Starting from the center, first heights H(i) and then widths D(i) of trapesoidal cells are drawn. Please note that sloppy work easily leads to forming distorted 3D parabolas. Fortunately, precision better than 1 mm = 1/25 inch is not hard to achieve on most suitable materials. Mentioning materials, it is up to the designer to figure out what suits their own needs. For example, LP found out that simple packaging paperboard treated with some lackeur or paint works amazingly well: it is rigid enough, costs nothing, and, the most important of all should one plan to hold the reflector by hands - weighs practically nothihg.

Technically inclined readers might be interested in mathematical formulas this calculator is based upon. As can be seen further below, the algorithm is iterative in nature and fairly complicated at first sight. Perhaps it could be simplified a bit or some more exact method could be used instead. Nevertheless, it makes its creator very happy and stisfied. The reason is that the algorithm was concieved in his scratchbook out of desperation on otherwise beutiful Serbian spring afternoon after he got extremely frustrated by inability to google out any suitable practical method for calculating parabolic dishes. A few hours after the initial misfortune struck, this geometrical method emerged. It still works pretty well - a nice feature of mathematics is that it is eternal by its nature.